Measuring specific charge of an electron

A purposeof the work isto study the dependence of anode current of electron tube on solenoid current Ia = f(IL); define the specific charge of electron e/m.

Instrumentation and appliances:electron tube, solenoid, microаmmeter, milliammeter, voltmeter, source of current.

Theoretical part

Fundamental characteristics of the electron are charge and mass. Relation of the charge of the electron to its mass is a specific charge of the electron:

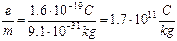
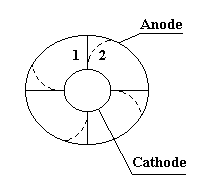This characteristic of the electron we can obtain by a magnetron method. This method makes use of the influence of a magnetic field on the electron that moves in a radial electric field. The electron tube is in the magnetic field of a solenoid. The electric field is perpendicular to the magnetic one. The electrons in the electron tube move from the cathode to the anode along a radius (1) if the magnetic field is absent (Fig.15.1).

Lorenz’s force acting on the electrons when they move in the magnetic field of the solenoid is 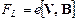, and therefore trajectory of motion will be distorted (curve 2 in the Fig.15.1).

The trajectory of the electron will be closed when intensity of the

Figure 15.1 magnetic field is quit sufficiently high. In this case the specific charge of the electron is defined as:

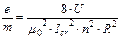, (15.1)

where U - potential difference of the cathode and anode; m₀ - magnetic constant; I_cr - critical current of the solenoid; n - quantity of turns per unit of the solenoid length; R - radius of solenoid.

Experimental part

The wiring scheme is shown in Fig. 15.2:

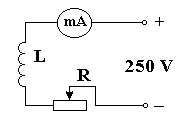
Figure 15.2

When current doesn't flow through the solenoid the anode current of the electron tube will be maximum. If current begins to flow through the solenoid and increases the anode current will decrease.

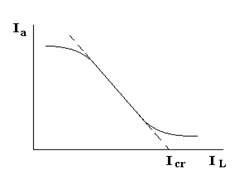
The anode current equal to zero corresponds to I_cr (Fig. 15.3).

2. The handle of voltage control is in the left position of the front panel of the current source ВУП-2. This handle must be in the position "0" when the source of current is turned off.

3. Switch on the source of current.

4. Write the value of the anode current I_a and voltage U when the solenoid current is absent and when the electron tube is being heated.

5. Increase the solenoid current from "0" to max value and write down the values of the anode current in the table.

6. Plot the graph of I_a = f(I_L).

Figure 15.3

7. Define the I_cr on this graph.

8. Calculate e/m by the formula (15.1), where m₀ = 4p × 10^-7 H/m,

n = 5 × 10⁴ 1/m, R = 5 × 10^-3 m.

This instruction is worked out by S. Lushchin, reader of the physics chair.

Reviewer: S. Loskutov, professor of the physics chair.